Page 59 - needle bearings

Technical Data
NTN

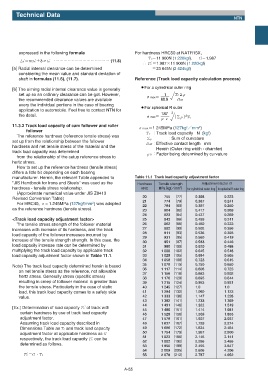
expressed in the following formula For hardness HRC50 at NATR15X,
Tcʹ11 900N (1 220kgf)ɼ Gʹ1.987
Li ʹmLi ʶ3МLi ʜʜʜʜʜʜʜʜʜʜʜʜʜʜ(11.8)
ˀ Tc ʹ1.987ʷ11 900N (1 220kgf)
[5] Radial internal clearance can be determined ʹ23 645N (2 424kgf)
considering the mean value and standard deviation of
shaft in formulas (11.6), (11.7). Reference (Track load capacity calculation process)

¡For a cylindrical outer ring
[6] The aiming radial internal clearance value is generally
set up so an ordinary clearance can be got. However, 1 TcЄЛ
ɹМmaxʹ
the recommended clearance values are available 60.9 Beff
every the individual portions in the case of bearing
¡For spherical R outer
application to automobile. Feel free to contact NTN for 3
the detail. ɹМmaxʹ 187 2
ЖЗ ʢЄЛʣTc
11.3.2 Track load capacity of cam follower and roller
2
МNBYʹ1 245MPa (127kgfʗmm )
follower Tc ɿTrack load capacity N (kgf)
The reference hardness (reference tensile stress) was
ЄЛ ɿSum of curvature
set up from the relationship between the follower BeffɿEffective contact length mm
hardness and net tensile stress of the material and the
Herein (Outer ring width - chamfer)
track load capacity was determined ЖЗɿFactor being determined by curvature
from the relationship of the setup reference stress to
hertz stress.
How to set up the reference hardness (tensile stress)
differs a little bit depending on each bearing
manufacturer. Herein, the relevant Table appended to Table 11.1 Track load capacity adjustment factor
"JIS Handbook for Irons and Steels" was used as the Hardness Tensile strength Adjustment factor G
hardness - tensile stress relationship. HRC MPaʨkgfʗmm ʩ for cylindrical outer ring for spherical R outer ring
2

(Approximate numerical value under JIS Z8413
Revised Conversion Table) 20 755 ʨ77ʩ 0.368 0.223
0.387
774
ʨ79ʩ
21
0.241
2
For HRC40, М= 1.245MPa (127kgf/mm ) was adopted 22 784 ʨ80ʩ 0.397 0.250
as the reference hardness (tensile stress). 23 804 ʨ82ʩ 0.417 0.269
24 823 ʨ84ʩ 0.437 0.289
<Track load capacity adjustment factor> 25 843 ʨ86ʩ 0.459 0.311
The tensile stress strength of the follower material 26 862 ʨ88ʩ 0.480 0.333
increases with increase of its hardness, and the track 27 882 ʨ90ʩ 0.502 0.356
28 911 ʨ93ʩ 0.536 0.393
load capacity of the follower increases incurred by 29 931 ʨ95ʩ 0.560 0.419
increase of the tensile strength strength. In this case, the
30 951 ʨ97ʩ 0.583 0.446
load capacity increase rate can be determined by 31 980 ʨ100ʩ 0.620 0.488
multiplying the track load capacity by applicable track 32 1 000 ʨ102ʩ 0.645 0.518
load capacity adjustment factor shown in Table 11.1. 33 1 029 ʨ105ʩ 0.684 0.565
34 1 058 ʨ108ʩ 0.723 0.615
Note) The track load capacity determined herein is based 35 1 078 ʨ110ʩ 0.750 0.650
on net tensile stress as the reference, not allowable 36 1 117 ʨ114ʩ 0.806 0.723
37 1 156 ʨ118ʩ 0.863 0.802
hertz stress. Generally stress (specific stress) 38 1 176 ʨ120ʩ 0.893 0.844
resulting in creep of follower material is greater than 39 1 215 ʨ124ʩ 0.953 0.931
the tensile stress. Particularly in the case of static 40 1 245 ʨ127ʩ 1.0 1.0
load, this track load capacity comes to a safety side 41 1 294 ʨ132ʩ 1.080 1.123
value. 42 1 333 ʨ136ʩ 1.147 1.228
43 1 382 ʨ141ʩ 1.233 1.369
44 1 431 ʨ146ʩ 1.322 1.519
[Ex.] Determination of load capacity Tc of track with 45 1 480 ʨ151ʩ 1.414 1.681
certain hardness by use of track load capacity 46 1 529 ʨ156ʩ 1.509 1.853
adjustment factor. 47 1 578 ʨ161ʩ 1.607 2.037
Assuming track load capacity described in 48 1 637 ʨ167ʩ 1.729 2.274
Dimensions Table as Tc and track load capacity 49 1 686 ʨ172ʩ 1.834 2.484
adjustment factor at applicable hardness as G 50 1 754 ʨ179ʩ 1.987 2.800
51 1 823 ʨ186ʩ 2.145 3.141
respectively, the track load capacity Tc can be 52 1 882 ʨ192ʩ 2.286 3.455
determined as follows.
53 1 950 ʨ199ʩ 2.455 3.847
54 2 009 ʨ205ʩ 2.606 4.206
Tc ʹGɾTc 55 2 078 ʨ212ʩ 2.787 4.652

A-55